Chaos Deconstruction: Double Snake

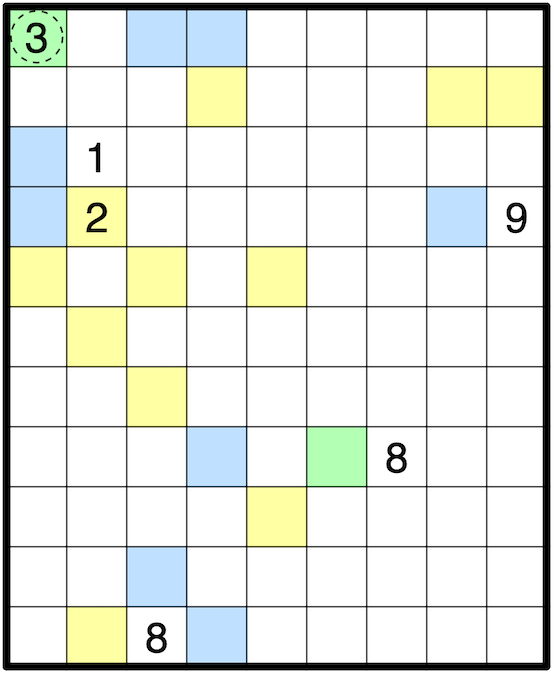
Rules:

Place the numbers from 1 to 9 in the grid such that no number repeats in any row or column. Some numbers are already given. Each number belongs to an orthogonally connected region that contains each number from 1 to 9 exactly once. The regions must be located by the solver, and there are 9 total regions that must be located.

Two snakes, we call them snake A and snake B, are to be drawn in the grid. A snake is a one cell wide path that travels orthogonally from cell center to cell center. The snakes must not touch themselves anywhere - orthogonally or diagonally, but they may touch or even intersect each other. However, no two adjacent cells may belong to both snakes. The dashed circle indicates an end of a snake.

A number in a yellow cell indicates how many of the cells in the surrounding 3x3 area belong to snake A. A number in a blue cell indicates how many of the cells in the surrounding 3x3 area belong to snake B. A green cell behaves as both a yellow cell and a blue cell. All colored cells are nonempty.

Every region is entirely part of snake A, or entirely part of snake B. Cells where the snakes intersect belong to two regions simultaneously.